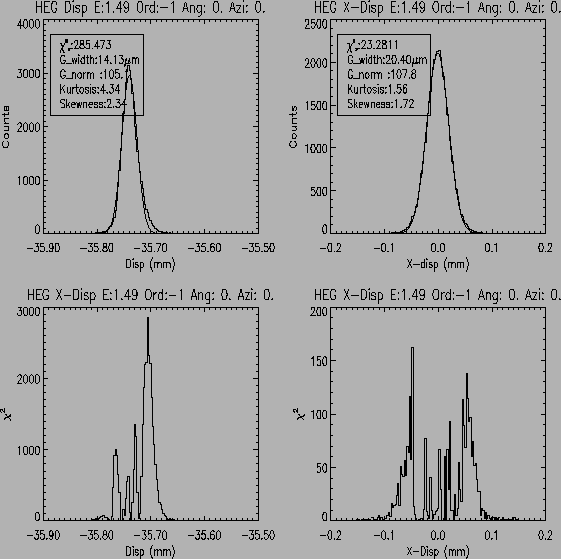
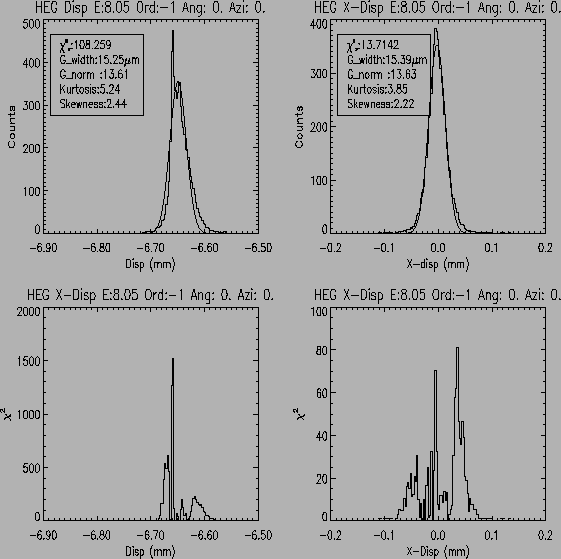
The LSF's are extracted from the simulations in the dispersion and
cross-dispersion directions using the same 5.3
![]() region
used for the library files. These profiles are then fit with a
Gaussian function with the center, width, and normalization free to
vary. These fits are done using the IDL fitting routine curvefit. In
general the Gaussian fits the central part of the line profile
reasonably well but the outer parts of the profile, the wings, are
systematically broader than a Gaussian (see figures
6,7). This can be seen in the
fits and the
region
used for the library files. These profiles are then fit with a
Gaussian function with the center, width, and normalization free to
vary. These fits are done using the IDL fitting routine curvefit. In
general the Gaussian fits the central part of the line profile
reasonably well but the outer parts of the profile, the wings, are
systematically broader than a Gaussian (see figures
6,7). This can be seen in the
fits and the ![]() plots where very large values of
plots where very large values of ![]() are
seen in the outer regions. The absolute value of
are
seen in the outer regions. The absolute value of ![]() is
determined using only statistical errors of the simulation and ignore
any calibration uncertainties for this calculation.
is
determined using only statistical errors of the simulation and ignore
any calibration uncertainties for this calculation.
|
|
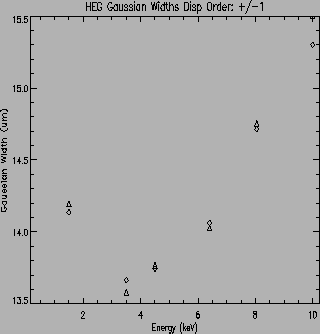
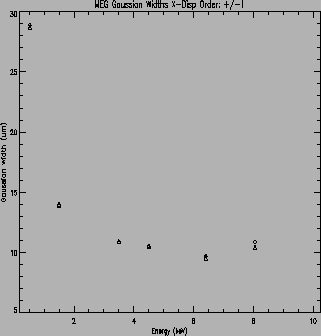
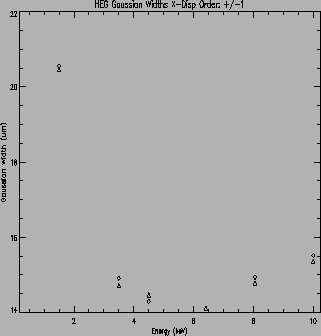
Plots of the Gaussian width vs Energy are given in
Figure 8, 9,
10. The HEG and MEG data show clear
trends of the Gaussian width with energy. The lowest energy (largest
dispersion distance) shows the largest width which rapidly decreases
to a fairly constant width for the MEG data. The HEG widths also
decrease rapidly with increasing energy, show a minimum width at
![]() 6.4 keV, and then shows a mild increase up to
6.4 keV, and then shows a mild increase up to ![]() 10 keV.
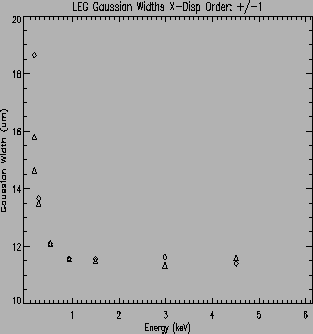
The LETG widths (figure 10) show a steap
decrease with increasing energy up to about 1 keV above which the
widths are fairly constant.
10 keV.
The LETG widths (figure 10) show a steap
decrease with increasing energy up to about 1 keV above which the
widths are fairly constant.
Table 3.3.1 shows the resolution (
![]() )
(as defined in HETG Ground Calibration: version 2.0 where
)
(as defined in HETG Ground Calibration: version 2.0 where
![]() )
for some of the measured lines shown in figure 8.
Not surprisingly, these values agree well with pre-flight design predictions and
with earlier predictions (see
http://space.mit.edu.ezproxyberklee.flo.org/HETG/res_power/res_power.html).
)
for some of the measured lines shown in figure 8.
Not surprisingly, these values agree well with pre-flight design predictions and
with earlier predictions (see
http://space.mit.edu.ezproxyberklee.flo.org/HETG/res_power/res_power.html).
| MEG | HEG | LEG | ||||
| Wavelength |
|
|
|
|||
| Å | ||||||
| 67.71 | |
|
|
|
11.70 | 1987 |
| 44.73 | |
|
|
|
15.20 | 1010 |
| 23.42 | 28.8 | 1570 | |
|
12.62 | 642 |
| 13.32 | |
|
|
|
11.93 | 383 |
| 8.32 | 14. | 767 | 20.5 | 991 | 11.74 | 242 |
| 3.54 | 10.9 | 352 | 14.8 | 443 | |
|
| 2.75 | 10.5 | 266 | 14.4 | 340 | |
|
| 1.94 | 9.65 | 196 | 14.1 | 234 | 11.74 | 122 |
| 1.54 | 10.6 | 146 | 14.9 | 177 | |
|
| 1.24 | |
|
15.5 | 136 | |
|

|
|

|